Open Journal of Chemistry
ISSN: 2618-0758 (Online) 2618-074X (Print)
DOI: 10.30538/psrp-ojc2018.0004
Zagreb Polynomials and redefined Zagreb indices of line graph of \(HAC_{5}C_{6}C_{7}[p,q]\) Nanotube
Aziz ur Rehman\(^1\), Waseem Khalid
Department of Mathematics, The University of Lahore (Pakpattan Campus), Lahore Pakistan.; (A.U. R & W.K)
\(^{1}\)Corresponding Author; rehmanmsc2014@gmail.com
Abstract
Keywords:
1. Introduction
Graph theory provides chemists with a variety of useful tools, such as topological indices. Molecular compounds are often modeled using molecular graphs. The molecular graph represents the structural formula of the compound in the form of graph theory, the vertices of which correspond to the atoms of the compound and the edges correspond to the chemical bonds [1].
Cheminformatics is a new area of research that integrates chemistry, mathematics, and information science. It studies the quantitative structure-activity (QSAR) and structure-property (QSPR) relationships [2, 3, 4, 5] used to predict the biological activity and properties of compounds. In the QSAR/QSPR study, the physical and chemical properties and topological indices such as Szeged index, Wiener index, Randić index, ABC index and Zagreb index etc were used to predict the biological activity of compounds. A molecular graph can be identified by topological index, polynomials, sequences or matrices [6].
The topological index is a number associated with the graph [7]. It represents the topological structure of the graph and is invariant under the automorphism of the graph. There are some major topological index categories, such as distance-based topological indices [8, 9], degree-based topological indices [10, 11], and counting-related polynomial and graph indices [7]. In these categories, the degree-based topological index is very important and plays a crucial role in chemical graph theory, especially in chemistry [12, 13, 14, 15]. More precisely, the topological index Top(G) of the graph is a number with the following characteristics: If a graph \(H\) is isomorphic to \(G\), then \(Top(H)=Top(G)\). The concept of topological index comes from Wiener [16], when he studied the boiling point of paraffin. He named this index as the path number. Later, the path number was renamed Wiener index.
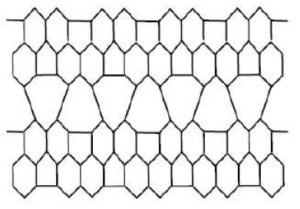
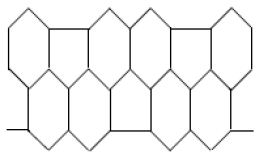
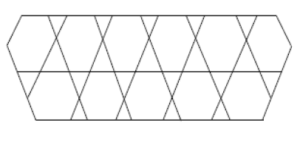
Carbon nanotubes form an interesting class of non-carbon materials [17]. There are three types of nanotubes, namely chiral, zigzag and armchairs nanotubes [18]. These carbon nanotubes show significant mechanical properties [17]. Experimental studies have shown that they belong to the most rigid and elastic known materials [19]. Diudea [20] was the first chemist to consider the topology of nanostructures. \(HAC_{5}C_{6}C_{7}[p,q]\) [21]shown in Figure 1, is constructed by alternating \(C_{5}\), \(C_{6}\) and \(C_{7}\) carbon cycles. It is tube shaped material but we consider it in the form of sheet shown in Figure 2. The two dimensional lattice of \(HAC_{5}C_{6}C_{7}[p,q]\) consists of \(p\) rows and \(q\) periods. Here \(p\) denotes the number of pentagons in one row and \(q\) is the number of periods in whole lattice. A period consist of three rows (See references [22, 23]). Figures are taken from [24]. Figure 3 is 2D graph of \(HAC_{5}C_{6}C_{7}[p,q]\) and Figure 4 is line graph of \(HAC_{5}C_{6}C_{7}[p,q]\).
Figure 1. \(HAC_{5}C_{6}C_{7}[p,q]\) Nanotube.
Figure 2. 2D graph of \(HAC_{5}C_{6}C_{7}[p,q]\).
Figure 3. \(HAC_{5}C_{6}C_{7}\).
Figure 4. \(L(HAC_{5}C_{6}C_{7})\)
The aim of this paper is to compute Zagreb polynomials of the line graph of \(HAC_{5}C_{6}C_{7}[p,q]\) nanotube. We also compute some degree-based topological indices of the line graph of \(HAC_{5}C_{6}C_{7}[p,q]\) nanotube. A line graph has many useful applications in physical chemistry [25, 26] and is defined as: the line graph \(L(G)\) of a graph \(G\) is the graph each of whose vertex represents an edge of \(G\) and two of its vertices are adjacent if their corresponding edges are adjacent in \(G\).
2. Basic definitions and Literature Review
Throughout this article, we take \(G\) as a connected graph, \(V(G)\) is the vertex set and \(E(G)\) is the edge set. The degree of a vertex \(v\) is denoted by \(d_{v}\) and is eqult to the number of vertics attached to \(v\).In the past two decades, a large number of topological indices have been defined and used for correlation analysis in theoretical chemistry, pharmacology, toxicology and environmental chemistry.
The first and second Zagreb indices are one of the oldest and most well-known topological indices defined by Gutman in 1972 and are given different names in the literature, such as the Zagreb group index, Sag. Loeb group parameters and the most common Zagreb index. The Zagreb index is one of the first indices introduced and has been used to study molecular complexity, chirality, ZE isomers and heterogeneous systems. The Zagreb index shows the potential applicability of deriving multiple linear regression models.
The first and the second Zagreb indices [27] are defined as \begin{equation*} M_{1}(G)=\prod\limits_{u\in V(G)}(d_{u}+d_{v}), \end{equation*} \begin{equation*} M_{2}(G)=\prod\limits_{uv\in E(G)}d_{u}\times d_{u}. \end{equation*} For details see [28]. Considering the Zagreb indices, Fath-Tabar ([29]) defined first and the second Zagreb polynomials as $$M_{1}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}+d_{v}},$$ and $$M_{2}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}.d_{v}}.$$ The properties of \(M_{1}(G,x)\) and \(M_{2}(G,x)\) for some chemical structures have been studied in the literature [30, 31].
After that, in [32], the authors defined the third Zagreb index $$M_{3}(G)=\sum\limits_{uv\in E(G)}(d_{u}-d_{v}),$$ and the polynomial $$M_{3}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}-d_{v}}.$$ In the year 2016, [33] following Zagreb type polynomials were defined $$M_{4}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{u}(d_{u}+d_{v})},$$ $$M_{5}(G,x)=\sum\limits_{uv\in E(G)}x^{d_{v}(d_{u}+d_{v})},$$ $$M_{a,b}(G,x)=\sum\limits_{uv\in E(G)}x^{ad_{u}+bd_{v}},$$ $$M'_{a,b}(G,x)=\sum\limits_{uv\in E(G)}x^{(d_{u}+a)(d_{v}+b)}.$$ Ranjini et al. [34] redefined the Zagreb index, i.e, the redefined first, second and third Zagreb indices of graph \(G\). These indicators appear as $$Re ZG_{1}(G)=\sum\limits_{uv\in E(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}},$$ $$Re ZG_{2}(G)=\sum\limits_{uv\in E(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}},$$ and $$Re ZG_{3}(G)=\sum\limits_{uv\in E(G)}(d_{u}+d_{v})(d_{u}.d_{v}). $$ For details about topological indices and its applications we refer [35, 36, 37, 38, 39, 40, 41, 42, 43].
3. Main Results
In this section, we present our computational results.Theorem 3.1. Let \(G\) be the line graph of \(HAC_{5}C_{6}C_{7}[p,q]\) nanotube. Then
- \(M_{3}(G,x)=2(38p-17)+2(6p+11)x,\)
- \(M_{4}(G,x)=2x^{8}+12x^{12}+(6p+1)x^{18}+(12p+10)x^{21}+(70p-37)x^{32},\)
- \(M_{5}(G,x)=2x^{8}+12x^{15}+(6p+1)x^{18}+(12p+10)x^{28}+(70p-37)x^{32},\)
- \(M_{a,b}(G,x)=2x^{2(a+b)}+12x^{2a+3b}+(6p+1)x^{3(a+b)}+(12p+10)x^{3a+4b}\\ +(70p-37)x^{4(a+b)},\)
- \(M'_{a,b}(G,x)=2x^{(a+2)(b+2)}+12x^{(a+2)(b+3)}+(6p+1)x^{(a+3)(b+3)}\\ +(12p+10)x^{(a+3)(b+4)}+(70p-37)x^{(a+4)(b+4)}.\)
Proof. Let \(G\) be the line graph of \(HAC_{5}C_{6}C_{7}[p,q]\) nanotube where \(p\) denotes the number of pentagons in one row and \(q\) denotes the number of periods in whole lattice. The edge set of line graph of \(HAC_{5}C_{6}C_{7}[p,q]\) with \(p\geq 1\) and \(q=2\) has following five partitions, $$E_{1}=E_{2,2}=\{e=uv\in E(HAC_{5}C_{6}C_{7}[p,q]): d_{u}=2, d_{v}=2\},$$ $$E_{2}=E_{2,3}=\{e=uv\in E(HAC_{5}C_{6}C_{7}[p,q]): d_{u}=2, d_{v}=3\},$$ $$E_{3}=E_{3,3}=\{e=uv\in E(HAC_{5}C_{6}C_{7}[p,q]): d_{u}=3, d_{v}=3\},$$ $$E_{4}=E_{3,4}=\{e=uv\in E(HAC_{5}C_{6}C_{7}[p,q]): d_{u}=3, d_{v}=4\},$$ and $$E_{5}=E_{4,4}=\{e=uv\in E(HAC_{5}C_{6}C_{7}[p,q]): d_{u}=4, d_{v}=4\}.$$ Such that $$\mid E_{1}(G)\mid=2,$$ $$\mid E_{2}(G)\mid=12,$$ $$\mid E_{3}(G)\mid=6p+1,$$ $$\mid E_{4}(G)\mid=12p+10,$$ $$\mid E_{5}(G)\mid=70p+37.$$
-
(1) \begin{eqnarray*}
M_{3}(G,x)&=& \sum\limits_{uv\in E(G)}x^{d_{u}-d_{v}}\\
&=&\sum\limits_{uv\in E_{1}(G)}x^{2-2}+\sum\limits_{uv\in E_{2}(G)}x^{3-2}+\sum\limits_{uv\in E_{3}(G)}x^{3-3}+\sum\limits_{uv\in E_{4}(G)}x^{4-3}\\
&&\sum\limits_{uv\in E_{5}(G)}x^{4-4}\\
&=&\mid E_{1}(G)\mid +\mid E_{2}(G)\mid x+\mid E_{3}(G)\mid +\mid E_{4}(G)\mid x +\mid E_{5}(G)\mid\\
&=& 2+12x+(6p+1)+(12p+10)x+(70p-37)\\
&=& 2(38p-17)+2(6p+11)x.
\end{eqnarray*}
(2) \begin{eqnarray*}
M_{4}(G,x)&=&\sum\limits_{uv\in E(G)}x^{d_{u}(d_{u}+d_{v})}\\
&=&\sum\limits_{uv\in E_{1}(G)}x^{2(2+2)}+\sum\limits_{uv\in E_{2}(G)}x^{2(2+3)}+\sum\limits_{uv\in E_{3}(G)}x^{3(3+3)}+\sum\limits_{uv\in E_{4}(G)}x^{3(3+4)}\\
&&\sum\limits_{uv\in E_{5}(G)}x^{4(4+4)}\\
&=&\mid E_{1}(G)\mid x^{8}+\mid E_{2}(G)\mid x^{10}+\mid E_{3}(G)\mid x^{18}+\mid E_{4}(G)\mid x^{28} +\mid E_{5}(G)\mid x^{32}\\
&=& 2x^{8}+12x^{12}+(6p+1)x^{18}+(12p+10)x^{21}+(70p-37)x^{32}.
\end{eqnarray*}
(3) \begin{eqnarray*}
M_{5}(G,x)&=& \sum\limits_{uv\in E(G)}x^{d_{v}(d_{u}+d_{v})}\\
&=&\sum\limits_{uv\in E_{1}(G)}x^{2(2+2)}+\sum\limits_{uv\in E_{2}(G)}x^{3(3+2)}+\sum\limits_{uv\in E_{3}(G)}x^{3(3+3)}+\sum\limits_{uv\in E_{4}(G)}x^{4(4+3)}\\
&&\sum\limits_{uv\in E_{5}(G)}x^{4(4+4)}\\
&=&\mid E_{1}(G)\mid x^{8}+\mid E_{2}(G)\mid x^{15}+\mid E_{3}(G)\mid x^{18}+\mid E_{4}(G)\mid x^{28} +\mid E_{5}(G)\mid x^{32}\\
&=& 2x^{8}+12x^{15}+(6p+1)x^{18}+(12p+10)x^{28}+(70p-37)x^{32}.
\end{eqnarray*}
(4) \begin{eqnarray*}
M_{a,b}(G,x)&=&\sum\limits_{uv\in E(G)}x^{ad_{u}+bd_{v}}\\
&=&\sum\limits_{uv\in E_{1}(G)}x^{2a+2b}+\sum\limits_{uv\in E_{2}(G)}x^{2a+3b}+\sum\limits_{uv\in E_{3}(G)}x^{3a+3b}+\sum\limits_{uv\in E_{4}(G)}x^{3a+4b}\\
&&\sum\limits_{uv\in E_{5}(G)}x^{4a+4b}\\
&=&\mid E_{1}(G)\mid x^{2(a+b)} +\mid E_{2}(G)\mid x^{2a+3b}+\mid E_{3}(G)\mid ^{3(a+b)} +\mid E_{4}(G)\mid x^{3a+4b} \\
&&+\mid E_{5}(G)\mid x^{4(a+b)}\\
&=& 2x^{2(a+b)}+12x^{2a+3b}+(6p+1)x^{3(a+b)}+(12p+10)x^{3a+4b}\\
&&+(70p-37)x^{4(a+b)}.
\end{eqnarray*}
(5) \begin{eqnarray*}
M'_{a,b}(G,x)&=& \sum\limits_{uv\in E(G)}x^{(d_{u}+a)(d_{v}+b)}\\
&=&\sum\limits_{uv\in E_{1}(G)}x^{(2+a)+(2+b)}+\sum\limits_{uv\in E_{2}(G)}x^{(a+2)(3+b)}+\sum\limits_{uv\in E_{3}(G)}x^{(a+3)(3+b)}\\
&&+\sum\limits_{uv\in E_{4}(G)}x^{(3+a)(4+b)}+\sum\limits_{uv\in E_{5}(G)}x^{(4+a)+(4+b)}\\
&=&\mid E_{1}(G)\mid x^{(a+2)(b+2)} +\mid E_{2}(G)\mid x^{(a+2)(b+3)}+\mid E_{3}(G)\mid x^{(a+3)(b+3)}\\
&& +\mid E_{4}(G)\mid x^{(a+3)(b+4)} +\mid E_{5}(G)\mid x^{(a+4)(b+4)}\\
&=& 2x^{(a+2)(b+2)}+12x^{(a+2)(b+3)}+(6p+1)x^{(a+3)(b+3)}\\
&&+(12p+10)x^{(a+3)(b+4)}+(70p-37)x^{(a+4)(b+4)}.
\end{eqnarray*}
Theorem 3.2. For every \(p\geq 1\) and \(q=2\) consider \(G\) be the the graph of \(HAC_{5}C_{6}C_{7}[p,q]\) nanotube. Then
- \(Re ZG_{1}(G)=46p,\)
- \(Re ZG_{1}(G)= \frac{1187}{7}p+\frac{2727}{70}\),
- \(Re ZG_{1}(G)=2(5146p-1725)\).
Proof. From the edge partition of line graph of \(HAC_{5}C_{6}C_{7}[p,q]\) nanotube given in Theorem 3.1
-
(1)\begin{eqnarray*}
Re ZG_{1}(G)&=&\sum\limits_{uv\in E(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}}\\
&=&\sum\limits_{uv\in E_{1}(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}}+\sum\limits_{uv\in E_{2}(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}}+\sum\limits_{uv\in E_{3}(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}}\\
&&+\sum\limits_{uv\in E_{4}(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}}+\sum\limits_{uv\in E_{5}(G)}\frac{d_{u}+d_{v}}{d_{u}d_{v}}\\
&=&\mid E_{1}(G)\mid +\mid E_{2}(G)\mid \frac{5}{6}+\mid E_{3}(G)\mid \frac{6}{9}+\mid E_{4}(G)\mid \frac{7}{12} +\mid E_{5}(G)\mid\frac{8}{16}\\
&=&2 +(12)\frac{5}{6}+(6p+1)\frac{6}{9}+(12p+10) \frac{7}{12} +(70p+37)\frac{8}{16}\\
&=& 46p.
\end{eqnarray*}
(2)\begin{eqnarray*}
Re ZG_{2}(G)&=&\sum\limits_{uv\in E(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}}\\
&=&\sum\limits_{uv\in E_{1}(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}}+\sum\limits_{uv\in E_{2}(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}}+\sum\limits_{uv\in E_{3}(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}}\\
&&+\sum\limits_{uv\in E_{4}(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}}+\sum\limits_{uv\in E_{5}(G)}\frac{d_{u}.d_{v}}{d_{u}+d_{v}}\\
&=&\mid E_{1}(G)\mid +\mid E_{2}(G)\mid \frac{6}{5}+\mid E_{3}(G)\mid \frac{9}{6}+\mid E_{4}(G)\mid \frac{12}{7} +\mid E_{5}(G)\mid\frac{16}{8}\\
&=&2 +(12)\frac{6}{5}+(6p+1)\frac{9}{6}+(12p+10) \frac{12}{7} +(70p+37)\frac{16}{8}\\
&=& \frac{1187}{7}p+\frac{2727}{70}.
\end{eqnarray*}
(3) \begin{eqnarray*}
Re ZG_{2}(G)&=&\sum\limits_{uv\in E(G)}(d_{u}.d_{v})(d_{u}+d_{v})\\
&=&\sum\limits_{uv\in E_{1}(G)}(d_{u}.d_{v})(d_{u}+d_{v})+\sum\limits_{uv\in E_{2}(G)}(d_{u}.d_{v})(d_{u}+d_{v})\\
&&+\sum\limits_{uv\in E_{3}(G)}(d_{u}.d_{v})(d_{u}+d_{v})+\sum\limits_{uv\in E_{4}(G)}(d_{u}.d_{v})(d_{u}+d_{v})\\
&&+\sum\limits_{uv\in E_{5}(G)}(d_{u}.d_{v})(d_{u}+d_{v})\\
&=&16 \mid E_{1}(G)\mid +30 \mid E_{2}(G)\mid+54 \mid E_{3}(G)\mid + 84 \mid E_{4}(G)\mid +128 \mid E_{5}(G)\mid\\
&=&32 +30(12)+54(6p+1)+84 (12p+10)+128(70p+37)\\
&=& 2(5146p-1725).
\end{eqnarray*}
Competing Interests
The authors declare that they have no competing interests.References
- Devillers, J., & Balaban, A. T. (Eds.). (2000). Topological indices and related descriptors in QSAR and QSPAR. CRC Press. [Google Scholor]
- Karelson, M. (2000). Molecular descriptors in QSAR/QSPR. Wiley-Interscience. [Google Scholor]
- Karelson, M., Lobanov, V. S., & Katritzky, A. R. (1996). Quantum-chemical descriptors in QSAR/QSPR studies. Chemical reviews, 96(3), 1027-1044. [Google Scholor]
- Consonni, V., Todeschini, R., Pavan, M., & Gramatica, P. (2002). Structure/response correlations and similarity/diversity analysis by GETAWAY descriptors. 2. Application of the novel 3D molecular descriptors to QSAR/QSPR studies. Journal of chemical information and computer sciences, 42(3), 693-705. [Google Scholor]
- Ferguson, A. M., Heritage, T., Jonathon, P., Pack, S. E., Phillips, L., Rogan, J., & Snaith, P. J. (1997). EVA: A new theoretically based molecular descriptor for use in QSAR/QSPR analysis. Journal of computer-aided molecular design, 11(2), 143-152. [Google Scholor]
- Balaban, A. T., Motoc, I., Bonchev, D., & Mekenyan, O. (1983). Topological indices for structure-activity correlations. In Steric effects in drug design (pp. 21-55). Springer, Berlin, Heidelberg. [Google Scholor]
- Balaban, A. T. (1983). Topological indices based on topological distances in molecular graphs. Pure and Applied Chemistry, 55(2), 199-206. [Google Scholor]
- Balaban, A. T. (1982). Highly discriminating distance-based topological index. Chemical Physics Letters, 89(5), 399-404. [Google Scholor]
- Xu, K., Liu, M., Das, K. C., Gutman, I., & Furtula, B. (2014). A survey on graphs extremal with respect to distance-based topological indices. MATCH Commun. Math. Comput. Chem, 71(3), 461-508. [Google Scholor]
- Gutman, I. (2013). Degree-based topological indices. Croatica Chemica Acta, 86(4), 351-361. [Google Scholor]
- Furtula, B., Gutman, I., & Dehmer, M. (2013). On structure-sensitivity of degree-based topological indices. Applied Mathematics and Computation, 219(17), 8973-8978. [Google Scholor]
- Gutman, I., Furtula, B., & Elphick, C. (2014). Three new/old vertex-degree-based topological indices. MATCH Commun. Math. Comput. Chem, 72(3), 617-632. [Google Scholor]
- Rada, J., Cruz, R., & Gutman, I. (2014). Benzenoid systems with extremal vertex-degree-based topological indices. MATCH Commun. Math. Comput. Chem, 72(1), 125-136. [Google Scholor]
- Deng, H., Yang, J., & Xia, F. (2011). A general modeling of some vertex-degree based topological indices in benzenoid systems and phenylenes. Computers & Mathematics with Applications, 61(10), 3017-3023. [Google Scholor]
- Hayat, S., & Imran, M. (2015). On degree based topological indices of certain nanotubes. Journal of Computational and Theoretical Nanoscience, 12(8), 1599-1605. [Google Scholor]
- Wiener, H. (1947). Structural determination of paraffin boiling points. Journal of the American Chemical Society, 69(1), 17-20. [Google Scholor]
- Dresselhaus, G., & Riichiro, S. (1998). Physical properties of carbon nanotubes. World Scientific. [Google Scholor]
- Chae, H. G., Sreekumar, T. V., Uchida, T., \& Kumar, S. (2005). A comparison of reinforcement efficiency of various types of carbon nanotubes in polyacrylonitrile fiber. Polymer, 46(24), 10925-10935. [Google Scholor]
- Thostenson, E. T., Ren, Z., & Chou, T. W. (2001). Advances in the science and technology of carbon nanotubes and their composites: a review. Composites science and technology, 61(13), 1899-1912. [Google Scholor]
- Diudea, M. V., & Gutman, I. (1998). Wiener-type topological indices. Croatica Chemica Acta, 71(1), 21-51. [Google Scholor]
- Farahani, M. R. (2012). The first and second Zagreb indices, first and second Zagreb polynomials of \(HAC_{5}C_{6}C_{7}[p, q]\) And \(HAC_{5}C_{7}[p, q]\) nanotubes. International Journal of Nanoscience and Nanotechnology, 8(3), 175-180. [Google Scholor]
- Farahani, M. R. (2013). On the geometric-arithmetic and atom bond connectivity index of \(HAC_{5}C_{6}C_{7}[p, q]\) nanotube. hemical Physics Research Journal, 6(1), 21-26. [Google Scholor]
- Farahani, M. R. (2013). Atom bond connectivity and geometric-arithmetic indices Of \(HAC_{5}C_{6}C_{7}[p, q]\) nanotube. Int. J. Chemical Modeling, 5(1), 127-132. [Google Scholor]
- Yang, H., Sajjad, W., Baig, A. Q., \& Farahani, M. R. (2017). The Edge Version of Randic, Zagreb, Atom Bond Connectivity and Geometric-Arithmetic Indices of NA (Q)(P) Nanotube. International journal of advanced biotechnology and research, 8(2), 1582-1589. [Google Scholor]
- Gutman, I., & Estrada, E. (1996). Topological indices based on the line graph of the molecular graph. Journal of chemical information and computer sciences, 36(3), 541-543. [Google Scholor]
- Estrada, E., Guevara, N., & Gutman, I. (1998). Extension of edge connectivity index. Relationships to line graph indices and QSPR applications. Journal of chemical information and computer sciences, 38(3), 428-431. [Google Scholor]
- Das, K. C., Xu, K., & Nam, J. (2015). Zagreb indices of graphs. Frontiers of Mathematics in China, 10(3), 567-582. [Google Scholor]
- Gutman, I., & Das, K. C. (2004). The first Zagreb index 30 years after. MATCH Commun. Math. Comput. Chem., 50(1), 83-92. [Google Scholor]
- Fath-Tabar, G. H. (2011). Old and new Zagreb indices of graphs. MATCH Commun. Math. Comput. Chem.,, 65(1), 79-84. [Google Scholor]
- Ranjini, P. S., Lokesha, V., Bindusree, A. R., & Raju, M. P. (2012). New bounds on Zagreb indices and the Zagreb co-indices. Boletim da Sociedade Paranaense de Matemática, 31(1), 51-55. [Google Scholor]
- Fath-Tabar, G. (2009). Zagreb polynomial and pi indices of some nano structures. Digest Journal of Nanomaterials & Biostructures (DJNB), 4(1), 189-191. [Google Scholor]
- Bindusree, A. R., Cangul, I. N., Lokesha, V., & Cevik, A. S. (2016). Zagreb polynomials of three graph operators. Filomat, 30(7), 1979-1986. [Google Scholor]
- Ranjini, P. S., Lokesha, V., & Usha, A. (2013). Relation between phenylene and hexagonal squeeze using harmonic index. International Journal of Graph Theory, 1(4), 116-121. [Google Scholor]
- Alaeiyan, M., Farahani, M. R., & Jamil, M. K. (2016). Computation of the fifth geometric-arithmetic index for polycyclic aromatic hydrocarbons pahk. Applied Mathematics and Nonlinear Sciences, 1(1), 283-290. [Google Scholor]
- Jamil, M. K., Farahani, M. R., Imran, M., & Malik, M. A. (2016). Computing eccentric version of second Zagreb index of polycyclic aromatic hydrocarbons (PAHk). Applied Mathematics and Nonlinear Sciences, 1(1), 247-252. [Google Scholor]
- Farahani, M. R., Jamil, M. K., & Imran, M. (2016). Vertex PIv topological index of titania carbon nanotubes \(TiO2(m,n)\). Applied Mathematics and Nonlinear Sciences, 1(1), 175-182. [Google Scholor]
- Gao, W., & Farahani, M. R. (2016). Degree-based indices computation for special chemical molecular structures using edge dividing method. Applied Mathematics and Nonlinear Sciences, 1(1), 99-122. [Google Scholor]
- Basavanagoud, B., Gao, W., Patil, S., Desai, V. R., Mirajkar, K. G., & Pooja, B. (2017). Computing First Zagreb index and F-index of New C-products of Graphs. Applied Mathematics and Nonlinear Sciences, 2(1), 285-298. [Google Scholor]
- Lokesha, V., Deepika, T., Ranjini, P. S., & Cangul, I. N. (2017). Operations of Nanostructures via SDD, ABC\(_{4}\) and GA\(_{5}\) indices. Applied Mathematics and Nonlinear Sciences, 2(1), 173-180. [Google Scholor]
- Hosamani, S. M., Kulkarni, B. B., Boli, R. G., & Gadag, V. M. (2017). QSPR analysis of certain graph theocratical matrices and their corresponding energy. Applied Mathematics and Nonlinear Sciences, 2(1), 131-150. [Google Scholor]
- Sardar, M. S., Zafar, S., & Zahid, Z. (2017). Computing topological indices of the line graphs of Banana tree graph and Firecracker graph. Applied Mathematics and Nonlinear Sciences, 2(1), 83-92. [Google Scholor]
- Basavanagoud, B., Desai, V. R., & Patil, S. (2017). \((\beta,\alpha)\)-Connectivity Index of Graphs. Applied Mathematics and Nonlinear Sciences, 2(1), 21-30.
- Ramane, H. S., & Jummannaver, R. B. (2016). Note on forgotten topological index of chemical structure in drugs. Applied Mathematics and Nonlinear Sciences, 1(2), 369-374. [Google Scholor]