Open Journal of Mathematical Analysis
ISSN: 2616-8111 (Online) 2616-8103 (Print)
DOI: 10.30538/psrp-oma2019.0030
Small convective motions of a visco-elastic fluid filling completely a container when the fluid is heated from below
Abdelmalek Essaâdi University, Faculty of Sciences, M2SM ER28/FS/05, 93030 Tetuan, Morocco.; (H.E)
Université de Franche-Comté, 2B Rue des jardins, F – 25000, Besançon, France.; (P.C)
\(^{1}\)Corresponding Author: essaouini@yahoo.fr
Abstract
Keywords:
1. Introduction
The problem of the small motions of a layer of viscous fluid heated from below was the subject of numerous works that can be found in the book (chapter II,[1]). Some cases of a heated viscous fluid partially or completely filling a fixed container has been studied and discussed in the book [2].
In this paper, we consider a mass of visco-elastic fluid heated from below and filling completely a container, restricting to the more simple Oldroyd model [2]. We obtain the operatorial equations for the small motions of the fluid and for the heat transfert by using for the classical Boussinesq hypothesis [1, 2].
At first, we prove the existence and the symmetry of the spectrum and the stability of the system, if the coefficients of kinematic viscosity and temperature conductivity are sufficiently large. We show that there exists a set of positive real eigenvalues having a point on the real axis as point of accumulation and that the possible non real eigenvalues are in a suitable disk. Then, we prove that the problem can be reduced to the study of a Krein- Langer pencil [3], so that we obtain new results. In particular, there is only a finite number of non real eigenvalues. Finally, we obtain an existence and unicity theorem of the solution of the associated evolution problem by means of the semigroups theory.
2. The more simple Oldroyd model for a viscoelastic fluid
It is a matter of a viscoelastic fluid for which the tensor of viscous stresses \(\sigma'\) and the double tensor of deformation velocities \(\tau\) verify a differential relation [2]We set
In the following, we set
3. The Boussinesq approximation
When a viscous or viscoelastic fluid is nonuniformly heated, its density \(\tilde{\rho}(t,x)\) depends on modifications of the temperature field, that is, the deviation \(T(t,x)\) of the temperature from some mean value \(T_{\text{m}}=\text{constant}\). For small \(T(t,x)\), we admit that \[ \tilde{\rho}(t,x)=\rho \left[ 1-\delta T(t,x) \right] \] where \(\rho=\text{constant}\) is the density corresponding to \(T_{\text{m}}\) and \(\delta>0\) is the coefficient of thermal extension.In accordance with the Boussinesq approximation [1, 2], we admit that, in the equation of motion of the fluid, the density can be considered as constant, except in the external forces.
4. Position and equations of the problem
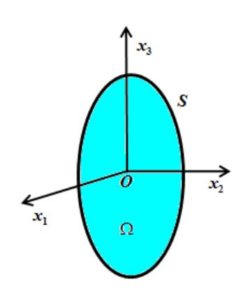
We introduce orthogonal axes of coordinates \(Ox_{1}x_{2}x_{3}\), \(Ox_{3}\) directed vertically upwards. The heavy viscoelastic fluid occupies a bounded domain \(\Omega\) with regular boundary \(S\) and \(g\) is the constant acceleration of the gravity. The fluid is nonuniformly heated from below.Figure 1. Model of the system.
If we take \[ \tau_{ij}=\frac{\partial u_{i}}{\partial x_{j}}+ \frac{\partial u_{j}}{\partial x_{i}} \qquad (i,j=1,2,3) \] the components of the tensor \(\tau\), we have the components of the tensor \(\sigma'\) \[ \sigma'_{ij}=\mu \hat{I}_{0}\tau_{ij} \] and then \[ \frac{\partial \sigma'_{ij}}{\partial x_{j}}= \mu \hat{I}_{0} \frac{\partial \tau_{ij}}{\partial x_{j}} =\mu \hat{I}_{0} \left( \frac{\partial^{2} u_{i}}{\partial x_{j} \partial x_{j}}+\frac{\partial}{\partial x_{i}} \left( \frac{\partial u_{j}}{\partial x_{j}} \right) \right)=\mu \hat{I}_{0} \Delta u_{i} \] since \(\frac{\partial u_{j}}{\partial x_{j}}=\text{div} \vec{u}=0\). Therefore, the vectorial equation of the motion of the fluid can be written
5. Conditions of mechanical equilibrium
There exist conditions of heating such that the system is in the state of mechanical equilibrium: \(\vec{u}=0\). (But, then, there is no thermodynamic equilibrium, the temperature varying with the coordinates).Setting \[ \vec{u}(t,x)=0;\qquad P=p_{0}(x);\qquad T=T_{0}(x), \] we obtain \[ \overrightarrow{\text{grad}}p_{0}=\rho g \delta T_{0} \vec{x}_{3};\qquad \Delta T_{0}=0 \] \(p_{0}\) and \(T_{0}\) are functions of \(x_{3}\) only and we have \[ \frac{\text{d}^2 T_{0}}{\text{d}x^{2}_{3}}=0 \] i.e \[ T_{0}=-\tilde{\alpha}x_{3}+\tilde{\alpha}_{0} \qquad (\tilde{\alpha}\;\;, \tilde{\alpha}_{0}\; \text{constants}). \] In the following, we consider the case \(\tilde{\alpha}>0\), corresponding to the heating from below.
The pressure in the state of mechanical equilibrium is given by \[ \frac{\text{d}p_{0}}{\text{d}x_{3}}=\rho g \delta(-\tilde{\alpha}x_{3}+\tilde{\alpha}_{0}) \] i.e \[ p_{0}(x_{3})=\rho g \delta \left(-\tilde{\alpha}\frac{x^{2}_{3}}{2}+\tilde{\alpha}_{0}x_{3} \right)+\text{constant} \] Concerning the boundary condition for the temperature, we suppose [2] that the temperature remain equal to \(T_{0}\) on the wall \(S\), so that, if we set \[ \theta(t,x)=T(t,x)-T_{0}(x_{3}) \] we have
6. Final formulation of the problem
Supposing the existence of the previous state of mechanical equilibrium, we seek the solutions of the equations in the form \[ \vec{u}=\vec{u}(t,x);\qquad P=p_{0}(x_{3})+p(t,x);\qquad T=T_{0}(x_{3})+ \theta(t,x) \] where \(\vec{u} \;, p\;, \theta\) are of the first order infinitesimal.We get
7. Transition to a system of operatorial equations
(1) We are going to seek \(\vec{u}(t,x),\; p(t,x)\), \(\theta (t,x)\) functions of \(t\) with values in \(J^{1}_{0}(\Omega)\), \(H^{1}(\Omega)\), \(H^{1}_{0}(\Omega)\) respectively, where \[ J^{1}_{0}(\Omega)=\left\{ \vec{u} \in \left[ H^1(\Omega)\right]^3;\;\; \text{div}\vec{u}=0 \;\; ; \;\; \vec{u}_{|S}=0 \right\} \] We introduce the space \[ J_{0}(\Omega)=\left\{ \vec{u} \in \mathcal{L}^2(\Omega) \overset{\text{def}}{=} \left[ L^2(\Omega)\right]^3;\;\; \text{div}\vec{u}=0\;\; ;\; u_{n|S}=0 \right\}, \] where \(u_{n|S}\) is the external normal component of \(\vec{u}\) on \(S\).\\ It is well-known [4] that the embedding from \(J^{1}_{0}(\Omega)\) into \(J_{0}(\Omega)\) is continuous, dense and compact and that we have the orthogonal decompositionWe have, for each \(\vec{\tilde{u}} \in J^{1}_{0}(\Omega) \):
The vectorial Laplacian formula [4] can be written by setting \( \epsilon_{ij}=\frac{1}{2}\tau_{ij}\) as \[ \int_\Omega \, \Delta {\vec{u}} \cdot \bar{\vec{\tilde{u}}} \, \mathrm d\Omega= -2\int_\Omega \,\epsilon_{ij}(\vec{u})\epsilon_{ij}(\bar{\vec{\tilde{u}}})+ 2\int_S \,\epsilon_{ij}(\vec{u})n_{j}\bar{\tilde{u}}_{i} \, \mathrm dS \] and the last integral is equal to zero by virtue of (11).
It is well-known that \[ \left( 2\int_\Omega \,\epsilon_{ij}(\vec{u})\epsilon_{ij}(\bar{\vec{u}}) \, \mathrm d\Omega \right)^{1/2} \] defines a norm on \(J^{1}_{0}(\Omega)\), that is equivalent to the classical norm of \( \left[ H^{1}(\Omega) \right]^{3}\).
We denote by \(A_{0}\) the unbounded operator of \(J_{0}(\Omega)\) associated to the pair \((J^{1}_{0}(\Omega),J_{0}(\Omega)) \) and to this norm.
Finally, denoting by \(P_{0}\), the orthogonal projector from \(\mathcal{L}^2(\Omega)\) into \(J_{0}(\Omega)\), we can write
\[ \left( \delta g \theta \vec{x}_{3},\vec{\tilde{u}} \right)_{\mathcal{L}^2(\Omega)}= \left(P_{0} ( \delta g \theta \vec{x}_{3}) ,\vec{\tilde{u}}\right)_{J_{0}(\Omega)} \] So, the variational equation (16) is equivalent to the operatorial equation [5]
As \[ \hat{I}_{0} A_{0}\vec{u}=A_{0}\vec{u}(t,x)+\alpha \int_{0}^{t} e^{-\gamma(t-s)}A_{0}\vec{u}(s,x) \, \mathrm ds \] By setting \[ \vec{u}_{0}(t,x)=\vec{u}(t,x); \qquad \vec{u}_{1}(t,x)= (\nu \alpha)^{1/2} \int_{0}^{t} e^{-\gamma(t-s)}A^{1/2}_{0}\vec{u}(s,x) \, \mathrm ds \] We have \[ (\nu \alpha)^{1/2} A^{1/2}_{0}\vec{u}(t,x)= \nu \alpha \int_{0}^{t} e^{-\gamma(t-s)}A_{0}\vec{u}(s,x) \, \mathrm ds \] and the equation (20) becomes
8. The normal oscillations
(1) We seek the solutions of the equations (22), (23), (21) in the form \[ \vec{u}_{0}=e^{-\lambda t}\vec{u}_{0}(x) \, ;\qquad \vec{u}_{1}=e^{-\lambda t}\vec{u}_{1}(x)\, ;\qquad w=e^{-\lambda t}w(x) \, ;\qquad \lambda \in \mathbb{C} \] We obtainIndeed, if \(\lambda =\gamma\), (25) gives \(\vec{u}_{0}=0\), then (26) gives $$A_{1}w=\frac{\gamma }{X}w $$and consequently \(w=0\), if dismiss the exceptional case where \(\frac{\gamma }{X}\) is an eigenvalue of \(A_{1}\); finally, (24) gives \(\vec{u}_{1}=0\). Therefore, we have \[ \vec{u}_{1}=-\frac{(\nu \alpha)^{1/2} A_{0}^{1/2}\vec{u}_{0}}{\lambda - \gamma} \] so that, taking (3) into account, the equation (24) takes the form \[ \nu I_{0}(\lambda)A_{0}\vec{u}_{0}-\epsilon P_{0}(w\vec{x}_{3})=\lambda \vec{u}_{0} \] Let us introduce the operators \(C\) from \(L^2(\Omega)\) into \(J_{0}(\Omega)\) and \(C^{\ast}\) from \(J_{0}(\Omega)\) into \(L^2(\Omega)\) defined by \[ Cw=P_{0}(w\vec{x}_{3}) \qquad;\qquad C^{\ast}\vec{u}=\vec{u}\cdot \vec{x}_{3} \] It is easy to verify that these operators are bounded, with a norm smaller that one, and mutually adjoints. Since \(\vec{u}_{0}=\vec{u}\), we obtain the equations of the normal oscillations in the form
Setting \[ D=A_{0}^{-1/2} C A_{1}^{-1/2};\qquad D^{\ast}=A_{1}^{-1/2} C^{\ast} A_{0}^{-1/2} \] we obtain
9. Existence and symmetry of the spectrum
We write the equations (29) and (30) in the form \[ \mathbf{J} \begin{pmatrix} \vec{U} \\ V \end{pmatrix} + \begin{pmatrix} 0 &-\epsilon D \\ -\epsilon D^{\ast} & 0 \end{pmatrix} \begin{pmatrix} \vec{U} \\ V \end{pmatrix} -\lambda \begin{pmatrix} A_{0}^{-1} &0 \\ 0 & A_{1}^{-1} \end{pmatrix} \begin{pmatrix} \vec{U} \\ V \end{pmatrix} =0 \] where \[ \mathbf{J}= \begin{pmatrix} \nu I_{0}(\lambda)I_{J_{0}(\Omega) } &0 \\ 0 & XI_{L^{2}(\Omega)} \end{pmatrix} \] has an inverse and \[ \begin{pmatrix} \vec{U} \\ V \end{pmatrix} \in \chi \overset{\text{def}}{=} J_{0}(\Omega)\oplus L^{2}(\Omega) \] Applying \(\mathbf{J}^{-1}\), we obtain \begin{equation*} \left\{ \begin{array}{l} I_{\chi} \begin{pmatrix} \vec{U} \\ V \end{pmatrix} + \begin{pmatrix} 0 &\nu^{-1} I_{0}(\lambda)^{-1}\epsilon D \\ -X^{-1}\epsilon D^{\ast} & 0 \end{pmatrix} \begin{pmatrix} \vec{U} \\ V \end{pmatrix}\\[.2cm] \\[.2cm] -\lambda \begin{pmatrix} \nu^{-1} I_{0}(\lambda)^{-1}A_{0}^{-1} &0 \\ 0 & X^{-1} A_{1}^{-1} \end{pmatrix} \begin{pmatrix} \vec{U} \\ V \end{pmatrix} =0 \\[.2cm] \end{array} \right. \end{equation*} that has the form \[ \left( I_{\chi} +\Phi (\lambda) \right)\begin{pmatrix} \vec{U} \\ V \end{pmatrix}=0 \] where \(\Phi(\lambda)\) is a operatorial function with compact values. Consequently, we obtain a Fredholm pencil in \(\mathbb{C}-\left\lbrace\beta \right\rbrace\), since \(\beta\) is a pole of \(I_{0}(\lambda)^{-1}\). This pencil is regular, then since \(\lambda=\gamma\) is not an eigenvalue, \(I+\Phi(\lambda)\) has a bounded inverse [4]. Then, the spectrum of the problem exists and consists of isolated points and its accumulation points may be located in \(\lambda=\beta\) and \(\lambda=\infty\). All points of the spectrum are eigenvalues and the corresponding eigenelements have finite multiplicities. On the other hand, it is obvious that the initial pencil is self adjoint, so that the spectrum is symmetrical with respect to the real axis.10. Location of the spectrum in the complex right half-plane
From the equations (29) and (30), we deduce11. Existence of a set of positive real eigenvalues having \(\lambda =\beta\) as point of accumulation
The equation (30) can be written as \[ \left( X I_{L^{2}(\Omega)}-\lambda A_{1}^{-1} \right)V+\epsilon D^{\ast} \vec{U}=0 \] \(A_{1}^{-1}\) has a denumerable infinity of positive real eigenvalues, the largest being \( \left\| A_{1}^{-1} \right\|\). Consequently, \( XI_{L^{2}(\Omega)}-\lambda A_{1}^{-1}\) has a bounded inverse if \(\lambda\) is not real and if \(\lambda\) is real with \(\left|\lambda\right|< \frac{X}{ \left\| A_{1}^{-1} \right\|}\). Under this condition, we have \[ V=-\epsilon \left(XI_{L^{2}(\Omega)}-\lambda A_{1}^{-1} \right)^{-1}D^{\ast}\vec{U} \] Carrying out in the equation (29), we obtain \[ \nu I_{0}(\lambda) \vec{U}+\epsilon^{2}D\left(XI_{L^{2}(\Omega)}-\lambda A_{1}^{-1} \right)^{-1}D^{\ast}\vec{U}-\lambda A_{0}^{-1}\vec{U}=0 \] If \(X\) is sufficiently large, the inequality \(\left|\lambda\right|< \frac{X}{ \left\| A_{1}^{-1} \right\|}\) is satisfied for \(\lambda=\beta\) and for \(\lambda\) sufficiently close to \(\beta\).Setting \[ \lambda=\lambda'+\beta,\qquad \left|\lambda'\right|\text{ sufficiently small} \] we obtain the equation
12. Location of the non real eigenvalues
We consider a non real eigenvalue \(\lambda\) and \( \begin{pmatrix} \vec{U} \\ V \end{pmatrix} \) an associated eigenelement.The equations (29) and (30) can be written \[ \left\{ \begin{array}{l} \nu \frac{I_{0}(\lambda)}{\lambda} \vec{U} - \frac{\epsilon}{\lambda} DV=A_{0}^{-1} \vec{U} \\[.2cm] \\[.2cm] -\frac{\epsilon}{\lambda}D^{\ast}\vec{U}+\frac{X}{\lambda}VI_{L^{2}(\Omega)}=A_{1}^{-1} V \\[.2cm] \end{array} \right. \] From these equations, we deduce \[ \left\{ \begin{array}{l} \nu \frac{I_{0}(\lambda)}{\lambda} \left\|\vec{U} \right\|_{J_{0}(\Omega)}^2 - \frac{\epsilon}{\lambda} \left(DV, \vec{U} \right)_{J_{0}(\Omega)}=\left(A_{0}^{-1} \vec{U}, \vec{U} \right)_{J_{0}(\Omega)} \\[.2cm] \\[.2cm] -\frac{\epsilon}{\bar{\lambda}}\left(DV, \vec{U} \right)_{J_{0}(\Omega)}+\frac{X}{\bar{\lambda}}\left\| V \right\|_{L^{2}(\Omega)}^2=\left(A_{1}^{-1} V, V \right)_{L^{2}(\Omega)} \\[.2cm] \end{array} \right. \] and then \[ \frac{\nu}{\epsilon} \Im \frac{I_{0}(\lambda)}{\lambda} \left\|\vec{U} \right\|_{J_{0}(\Omega)}^2 -\frac{X}{\epsilon}\Im \frac{1}{\bar{\lambda}} \left\| V \right\|_{L^{2}(\Omega)}^2 =-\frac{2 \Im \lambda}{\left|\lambda\right|^{2}} \Re \left(DV, \vec{U} \right)_{J_{0}(\Omega)} \] Calculating \( \Im \frac{I_{0}(\lambda)}{\lambda}\) and dividing by \(\Im \lambda \neq 0\), we obtain \[ \frac{\nu}{2}\left\|\vec{U} \right\|_{J_{0}(\Omega)}^2 \left[ \frac{\frac{\beta-\gamma}{\gamma}}{\left|\lambda-\gamma\right|^{2}}-\frac{\frac{\beta}{\gamma}}{\left|\lambda\right|^{2}} \right] -\frac{X}{\epsilon} \frac{1}{\left|\lambda\right|^{2}} \left\| V \right\|_{L^{2}(\Omega)}^2 = -\frac{2}{\left|\lambda\right|^{2}} \Re \left(DV, \vec{U} \right)_{J_{0}(\Omega)} \] or, using (32) \[ \left\{\frac{\nu}{2}\left[ \frac{\frac{\beta-\gamma}{\gamma}}{\left|\lambda-\gamma\right|^{2}}-\frac{\frac{\beta}{\gamma}}{\left|\lambda\right|^{2}} \right]+\frac{\left\| A_{0}^{-1} \right\|}{\left|\lambda\right|^{2}} \right\}\left\|\vec{U} \right\|_{J_{0}(\Omega)}^2 \geq \frac{1}{\left|\lambda\right|^{2}} \left[ \frac{X}{\epsilon}-\left\| A_{1}^{-1} \right\| \right]\left\| V \right\|_{L^{2}(\Omega)}^2 \] The right-hand side of above equation is positive according to (33), so that the eventual nonreal eigenvalues must verify
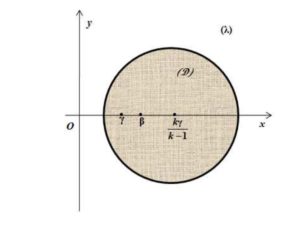
Setting \(\lambda=x+iy\), we see that the domain of the complex plane \((\lambda)\) defined by (35) is the disk \(\mathcal{D}\) defined by \[ \left(x-\frac{k\gamma}{k-1} \right)^2+y^{2}< \frac{k\gamma^{2}}{(k-1)^2} \] \(\lambda=\gamma\) belongs to \(\mathcal{D}\) and it is easy to verify that \(\lambda =\beta\) belongs to \(\mathcal{D}\).
Figure 2. Location of the non real eigenvalues.
13. Reduction to a Krein - Langer pencil
(1) Multiplying the equations 29) and (30) by \(\lambda - \gamma \neq 0\), we obtainWe have \[ \begin{aligned} &\left(\mathbf{\tilde{B}} z, z \right)_{\chi} = \nu \left\| \vec{U}\right\|^{2}_{J_{0}(\Omega)} +X\left\| V\right\|^{2}_{L^{2}(\Omega)} +\gamma \left[ \left(A_{0}^{-1} \vec{U}, \vec{U} \right)_{J_{0}(\Omega)} +\left(A_{1}^{-1} V, V \right)_{L^{2}(\Omega)} \right] \\ & \qquad \qquad \qquad -2\epsilon \Re \left(DV, \vec{U} \right)_{J_{0}(\Omega)} \end{aligned} \] Using the inequality (32), we obtain \[ \begin{aligned} &\left(\mathbf{\tilde{B}} z, z \right)_{\chi} \geq \left( \nu -\epsilon \left\| A_{0}^{-1} \right\| \right) \left\| \vec{U}\right\|^{2}_{J_{0}(\Omega)} + \left( X -\epsilon \left\| A_{1}^{-1} \right\| \right)\left\| V\right\|^{2}_{L^{2}(\Omega)} \\ & \qquad \qquad \qquad + \gamma \left[ \left(A_{0}^{-1} \vec{U}, \vec{U} \right)_{J_{0}(\Omega)} +\left(A_{1}^{-1} V, V \right)_{L^{2}(\Omega)} \right] \end{aligned} \] By virtue of the inequalities (33), \(\mathbf{\tilde{B}}\) is strongly positive. (c) \( \mathbf{Q}\) is bounded and self adjoint.
On the other hand, we have \[ \begin{aligned} &\left(\mathbf{Q} z, z \right)_{\chi} \geq \nu \left( \beta-\gamma \right)\left\| \vec{U}\right\|^{2}_{J_{0}(\Omega)} \\ & \qquad \qquad \qquad + \gamma \left[ \left( \nu -\epsilon \left\| A_{0}^{-1} \right\| \right) \left\| \vec{U}\right\|^{2}_{J_{0}(\Omega)} + \left( X -\epsilon \left\| A_{1}^{-1} \right\| \right)\left\| V\right\|^{2}_{L^{2}(\Omega)} \right] \end{aligned} \] so that \(\mathbf{Q}\) is strongly positive and then admits an inverse that has the same properties. (3) Setting \[ \mathbf{Q}^{1/2}z=W\in \chi \] carrying out in (38) and applying \(\mathbf{Q}^{-1/2}\), we obtain \[ W+\tilde{\lambda}\mathbf{Q}^{-1/2} \mathbf{\tilde{B}} \mathbf{Q}^{-1/2}W+ \tilde{\lambda}^{2}\mathbf{Q}^{-1/2} \mathcal{\tilde{C}} \mathbf{Q}^{-1/2}W=0 \] It is easy to see that \(\mathbf{B} =\mathbf{Q}^{-1/2} \mathbf{\tilde{B}} \mathbf{Q}^{-1/2}\) and \(\mathcal{C}=\mathbf{Q}^{-1/2} \mathcal{\tilde{C}} \mathbf{Q}^{-1/2}\) are bounded, self adjoint and positive definite and \(\mathcal{C}\) is compact.
So we obtain a Krein-Langer pencil [2; pp 295-309]
Since \(\mathbf{B}\) is self adjoint, the set of the nonreal eigenvalues may have only the infinity as point of accumulation.
Consequently, since these eigenvalues are in the disk \(\mathcal{D}\), there are no more than a finite number of such eigenvalues.
14. Existence and unicity of the solution of the associated evolution problem
We write the equations (22), (23), (21) in the form \[ \dot{\mathbf{W}}+\mathcal{A} \mathbf{W}=0 \] with \[ \mathbf{W}= \begin{pmatrix} \vec{u}_{0}\\ \vec{u}_{1} \\ w \end{pmatrix} \qquad , \quad \mathcal{A}= \begin{pmatrix} \nu A_{0} & (\nu \alpha)^{1/2}A^{1/2}_{0} & -\epsilon C \\ -(\nu \alpha)^{1/2}A^{1/2}_{0} & \gamma I_{J_{0}(\Omega)} & 0 \\ -\epsilon C^{\ast} & 0 & XA_{1} \end{pmatrix} \] We are going to consider \(\mathcal{A}\) as unbounded operator of \[ H=J_{0}(\Omega)\oplus J_{0}(\Omega) \oplus L^{2}(\Omega) \] with domain \[ D(\mathcal{A})=D(A_{0})\oplus D(A^{1/2}_{0}) \oplus D(A_{1}) \] We are going to prove that \(-\mathcal{A}\) is maximal dissipative. (1) \(\mathcal{A}\) is obviously closed.By direct calculations, we have \[ \begin{aligned} & \left(\mathcal{A} \mathbf{W}, \mathbf{W} \right)_{H}= \nu \left(A_{0} \vec{u}_{0}, \vec{u}_{0}\right)_{J_{0}(\Omega)} +\gamma \left\| \vec{u}_{1}\right\|^{2}_{J_{0}(\Omega)} +X \left(A_{1} w, w \right)_{L^{2}(\Omega)} -2\epsilon \Re \left(Cw, \vec{u}_{0}\right)_{J_{0}(\Omega)}\\ & \qquad \qquad \qquad +2i\Im \left(A^{1/2}_{0} \vec{u}_{1}, \vec{u}_{0}\right)_{J_{0}(\Omega)} \end{aligned} \] so that \[ \Re \left(\mathcal{A} \mathbf{W}, \mathbf{W} \right)_{H} \geq 0 \qquad \forall \mathbf{W}\in D(\mathcal{A}) \] under the condition (33). (2) We have \[ \mathcal{A}^{\ast}= \begin{pmatrix} \nu A_{0} & -(\nu \alpha)^{1/2}A^{1/2}_{0} & -\epsilon C \\ (\nu \alpha)^{1/2}A^{1/2}_{0} & \gamma I_{J_{0}(\Omega)} & 0 \\ -\epsilon C^{\ast} & 0 & XA_{1} \end{pmatrix} \] so that, in the same manner, we have \[ \Re \left(\mathcal{A}^{\ast} \mathbf{W}, \mathbf{W} \right)_{H} \geq 0 \qquad \forall \mathbf{W}\in D(\mathcal{A}^{\ast})=D(\mathcal{A}) \] Consequently, [3;p 54,55], if the initial value \(\mathbf{W}(0)\in D(\mathcal{A})\), i.e \[ \vec{u}_{0}(0)\in D(A_{0}) \qquad,\qquad \vec{u}_{1}(0)\in D(A^{1/2}_{0}) \qquad,\qquad w(0)\in D(A_{1}) \] the evolution problem has a solution and only one belonging to \(D(\mathcal{A})\) for \(t\geq 0\).
Acknowledgment
The authors are grateful to the referee and the editorial board for some useful comments that improved the presentation of the paper.Author Contributions
All authors contributed equally to the writing of this paper. All authors read and approved the final manuscript.Competing Interests
The author(s) do not have any competing interests in the manuscript.References
- Chandrasekhar, S. (2013). Hydrodynamic and hydromagnetic stability. Dover pubications, Inc. New York. [Google Scholor]
- Kopachevsky, N. D., & Krein, S. (2003). Operator Approach to Linear Problems of Hydrodynamics: Volume 2: Nonself-adjoint Problems for Viscous Fluids (Vol. 146). Birkhäuser.[Google Scholor]
- Israil'Cudikovic Gochberg, Krejn, M. G. E., & Roos, G. (1971). Introduction à la théorie des opérateurs linéaires non auto-adjoints dans un espace hilbertien. Dunod.[Google Scholor]
- Kopachevsky, N. D., & Krein, S. (2001). Operator Approach to Linear Problems of Hydrodynamics: Volume 1: Nonself-adjoint Problems for Viscous Fluids (Vol. 146). Birkhäuser.[Google Scholor]
- Lions, J. L. (2013). Equations differentielles operationnelles: et problémes aux limites (Vol. 111). Springer-Verlag.[Google Scholor]